This article was published in Scientific American’s former blog network and reflects the views of the author, not necessarily those of Scientific American
If you’ve doodled in math class, you might have stumbled on a Koch snowflake accidentally. You can make one by starting with an equilateral triangle. Then take six equilateral triangles each 1/3 the size of the original triangle and stick them to the middle thirds of each side. Now rinse and repeat. And repeat. And repeat, ad infinitum.
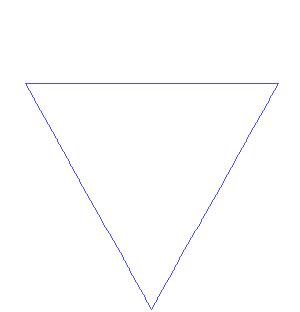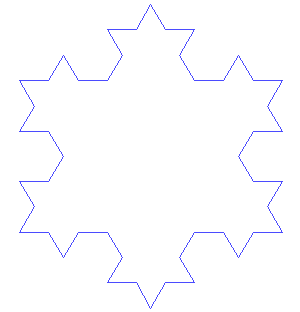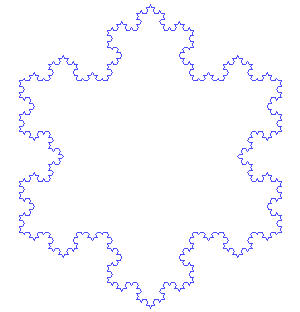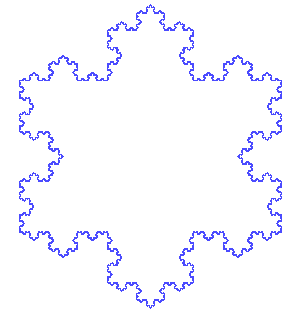
Several iterations in the construction of the Koch snowflake. Credit: António Miguel de Campos Wikimedia
Francophones (or middling Francophakers like myself) can read the 1904 paper by Helge von Koch in which the Koch curve first appears for free online. (The Koch curve is one side of the Koch snowflake; in other words, you can get a Koch snowflake by sticking three Koch curves together.) Von Koch invented the curve as a more intuitive and immediate example of a phenomenon Karl Weierstrass had documented decades before. It all comes down to tangents. Smooth curves have tangent lines—lines that touch a function at one point and point in the same direction the curve is going at that one particular spot.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
Before Weierstrass had discovered his “monsters,” some mathematicians assumed that if a function was continuous—no big jumps or gaps—it must have tangent lines almost everywhere. There might be an odd corner or cusp here or there, but they would be isolated. Basically, a function couldn’t be all corners. Weierstrass found counterexamples, but they were hard to understand and imagine. The Koch curve also has no tangents anywhere, but von Koch’s geometric construction makes it a lot easier to understand. Everywhere you add a spike, you’re adding a corner. And there are no segments without spikes in them. This example is much more tangible, and as a bonus, quite attractive atop a Sierpinski holiday tree.
Like many fractals, the Koch snowflake falls through the cracks of our normal conception of dimension. The boundary of the snowflake is not quite one-dimensional and not quite two-dimensional. It has infinite length, which you can see by noting that we multiply the total length of the curve by 4/3 in each iteration, and the product of infinitely many 4/3’s is not finite. But it has no area. (The boundary, that is. The interior of the Koch snowflake is two-dimensional and has a well-defined area.)
Serendipitously, as I was writing this post, my pal Katie Mann, a mathematician at Brown University, shared an important practical application of the Koch snowflake: the Koch pecan pie. On the Instructables website, Charless Fowlkes describes and documents his adventure making a giant Koch pecan pie. (If my back-of-the-envelope calculations are correct, it contains about 5 dozen eggs and 10 pounds of pecans!) He notes that if you make a traditional circular pie with a very large radius R, “the amount of filling scales as R2 while the crust only scales linearly so as the pie grows larger, the flaky crust is completely dominated by the creamy filling.” The crust of the Koch version, on the other hand, grows more quickly than linearly, allowing crust-lovers to have more crust with their pie.

The Koch pecan pie cooking on a purpose-built oven. Find more pictures of the Koch pie at Instructables and Google Photos. Credit: Charless Fowlkes
Folwkes reports that building the Koch pie tin out of metal helped him develop a more visceral understanding of what it means for a curve to have many points without tangents. He writes, "i mean, you think you have a pretty good grasp of the mathematical analysis but until a piece of metal with a very high perimeter to surface area ratio tears into your flesh, you're really missing an intuitive appreciation for objects that lack continuous derivatives almost everywhere."
The Koch snowflake pie was a noble endeavor, but I believe it could be improved. The crust, though its size scales nicely, is stuck out on the edges. With a pie this large, radial pie slices are unwieldy if not downright impossible, so people with interior pieces will still be forlorn and crustless.
But another property of the Koch snowflake can help. The snowflake tiles itself. If you take six smaller copies of the Koch snowflake and stick them in each arm, you’ll be left with another Koch snowflake in the middle. It’s larger than the ones in the arms, but it’s the same shape. Instead of leaving that inner snowflake alone, you could again tile it with smaller snowflakes, and so on.
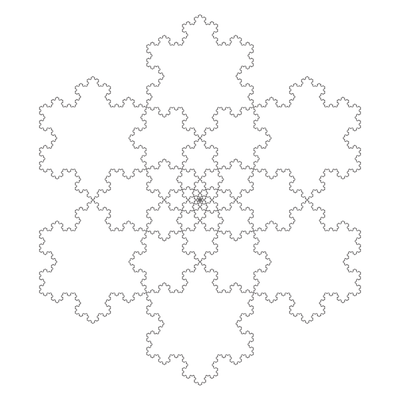
A tessellation of a Koch snowflake with smaller Koch snowflakes of many scales. Now imagine if those boundaries were made of delicious, flaky crust. Credit: Edmund Harriss Patterns of the Universe, a mathematical coloring book by Edmund Harriss and Alex Bellos
In pie form, this suggests that you can build fractal walls of crust throughout the pie, distributing it more evenly. In fact, if you tile that inner snowflake with smaller snowflakes, and the inner snowflakes of those snowflakes, and so on, you can satisfy even the crustiest pie-eaters.
Read about more of my favorite spaces: The Cantor Set Fat Cantor Sets The Topologist’s Sine Curve Cantor's Leaky Tent The Infinite Earring The Line with Two Origins The House with Two Rooms The Fano Plane The Torus The Three-Torus The Möbius Strip The Long Line Space-Filling Curves The Wallis Sieve Two Tori Glued along a Slit The Empty Set The Menger Sponge The Connected Sum of Four Hopf Links Borromean Rings The Sierpinski Triangle Lexicographic Ordering on the Unit Square The SNCF Metric The Mandelbrot Set Fatou's Pancake The Pseudosphere The Douady Rabbit The Poincaré Homology Sphere The Kovalevskaya Top A 6-Holed Torus The Real Projective Plane The 1-Dimensional Sphere The Loch Ness Monster
