This article was published in Scientific American’s former blog network and reflects the views of the author, not necessarily those of Scientific American
It’s an internationally recognized symbol of unity, healthy competition, athletic prowess, and the excellence of people named Simone. But on to the important part: is there anything mathematically interesting about the Olympic rings?
Yes and no. The Olympic rings symbol is a chain of five rings, each one attached to one or two neighbors. We’ll take a trip to the world of knot theory to figure out how to analyze it mathematically.
A mathematical knot is almost like a knot you would have in your shoelace or sailboat rigging, but the loose ends are fused together. A circle, then, is a knot, but it’s not a very compelling one. It, and anything you can get by jigging it around without cutting or gluing, is called the unknot.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.

An unknotted binder clip. Image: Evelyn Lamb
The technical term for an object like the Olympic rings is a link: it is a bunch of knots that interact with each other in some way. Two circles sitting next to each other, politely not touching, are technically a link, but once again not a very compelling one. They would be called trivially linked.

Unlinked unknots. Image: Evelyn Lamb
The simplest interesting link is the Hopf link: two interlocking circles.

A Hopf link. Image: Evelyn Lamb
The Olympic rings are kind of like the Hopf link, but longer. To some extent that makes them less interesting because it’s relatively easy to build them up from smaller pieces. To make that idea precise, we can define something called a prime link. (The definition of prime knot is similar, but we’ll just focus on links because the Olympic rings form a link.)
Prime numbers, those whole numbers greater than 1 that are divisible only by themselves and 1, can be thought of as the building blocks of all other whole numbers because you can break down a whole number into its prime factors. Similarly, links can be decomposed into prime links. Instead of defining prime link, it’s easier to define a link that isn’t prime. We do that using an operation called a connected (sometimes called connect) sum that describes how we can build new knots and links from old knots and links. To form the connected sum of two links, you cut a segment out of each of them and re-glue them so they’re glued together. A link that can’t be built from two or more non-trivial links is called prime.
To see how a connected sum works, we’ll look at how the connected sum of two Hopf links gives us a chain of three linked rings. In step 1, two Hopf links approach one another.

Step 1. Image: Evelyn Lamb
In step 2, they open up.

Step 2. Image: Evelyn Lamb
In step 3, the two open rings meet each other.

Step 3. Image: Evelyn Lamb
Now, the middle two rings, shown here in silver, are connected, so from a topological point of view, they have fused to become one ring. Hence in the final step, we replace those two rings with one ring that is topologically equivalent.
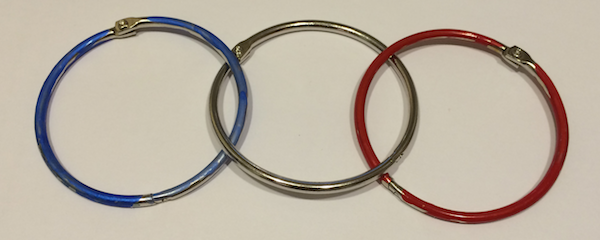
Step 4: Success! The two Hopf links have fused to become a chain of three rings. Image: Evelyn Lamb
You can keep connected summing Hopf links together to get as many rings in your link as you want. A similar link with n rings in it takes n-1 copies of the Hopf link to make, so the Olympic rings are a connected sum of four Hopf links.
It seems a bit disappointing that a symbol of the best of the human race is not a prime link. So I asked Jessica Purcell, a mathematician at Monash University in Australia, whether she had a recommendation for a better five-ring link so the IOC could adopt a mathematically more satisfying symbol if they are so inclined. She suggested the minimally twisted 5-chain link, which she describes as one of her favorites. That’s a mouthful, but a picture will help.

A minimally twisted 5-chain link. Image: Evelyn Lamb
Basically, you attach the two ends of the connected sum of four Hopf links together, and you've got it. You have to be a little careful to get the crossings exactly right, but it’s not too hard to do. (You can see a diagram of the link on page 2 of this paper.)
Why is the minimally twisted 5-chain link so great? Well, first of all it’s prime, so it stands on its own merits, unlike the current symbol, which is built up from smaller links. Another reason Purcell told me about is due to the relationship knots and links have to three-dimensional spaces, also known as three-manifolds. In fact, one of the reasons mathematicians study knots and links is not for their own sake but because of these spaces, called knot or link complements.
How do knots and links get tied up (har!) with three-manifolds? You take your knot or link, made out of string or binder rings or something, and imagine removing it from three-dimensional space*. Knot theorists sometimes call it "drilling it out" of the space. The remaining manifold is called the knot or link complement. For example, if you’re wearing a ring, you can think about the the ring's complement as being some three-dimensional space minus your ring. Topologists, who are often concerned with how many fundamentally different loops you can make in a space, find the space minus the ring more interesting than the space by itself. That’s because in the larger space, every loop is basically the same as every other loop. You can just scoot and stretch one loop over to another one without cutting or tearing anything.
For more complicated knots and links, the complements get even more interesting than the complement of a ring. Purcell told me she likes the minimally twisted 5-chain link in part because of its complement. If you want to study it geometrically, you can build it out of 10 shapes called regular ideal hyperbolic tetrahedra. That means that with a bit of poetic license, you could make it out of 10 copies of the Rio 2016 logo, which is topologically equivalent to the edges of a tetrahedron.
Purcell assures me that manifolds that can be built from regular ideal hyperbolic tetrahedra are very special indeed. Getting all the way into what that means is not going to happen in this blog post, but she has some lecture notes on hyperbolic knot theory if you want to go a bit further.
In case you were wondering, the complement of the minimally twisted 5-chain link is way better than the complement of the current Olympic rings, which is based on the complement of the Hopf link. The complement of the Hopf link has Euclidean geometry, or as I like to call it when I’m feeling disrespectful of thousands of years of mathematical tradition, snooze-clidean geometry. I’ll take hyperbolic geometry over Euclidean geometry any day!
*If you’re especially fastidious, you’ll probably want to point out that I told a small fib here. An infinite, unbounded three-manifold with a tiny knot removed is a bit unwieldy, so instead of starting with infinite three-dimensional space, mathematicians actually imagine removing it from something called the three-sphere, or S3. It’s similar to a circle or a sphere but one dimension higher.
If that’s confusing, don't worry about it too much. No one is going to report you to the math police if you think of removing it from regular three-dimensional space instead. There is a good reason mathematicians do it the way they do: it’s a lot easier to work with finite, or bounded, spaces than infinite ones. (Just like the two-dimensional sphere, or the surface of a beach ball, the three-sphere has a finite radius.) But as far as imagining a knot complement goes, I find it much easier to visualize if I think about removing the knot from regular three-dimensional space rather than the three-sphere.
Thanks to Dave Futer, Jessica Purcell, and Dylan Thurston for lending me some knot theory knowledge.
Read about more of my favorite spaces: The Cantor Set Fat Cantor Sets The Topologist’s Sine Curve Cantor's Leaky Tent The Infinite Earring The Line with Two Origins The House with Two Rooms The Fano Plane The Torus The Three-Torus The Möbius Strip The Long Line Space-Filling Curves The Wallis Sieve Two Tori Glued along a Slit The Empty Set The Menger Sponge
