This article was published in Scientific American’s former blog network and reflects the views of the author, not necessarily those of Scientific American
I must confess that I didn’t know about Cantor’s Leaky Tent until I wrote about the π-Base, a website that collects information about interesting mathematical spaces. It has such a delightfully whimsical name that I had to learn more about it. You might want to be familiar with the Cantor set before reading about the leaky tent—lucky for you, I wrote about it back in March.
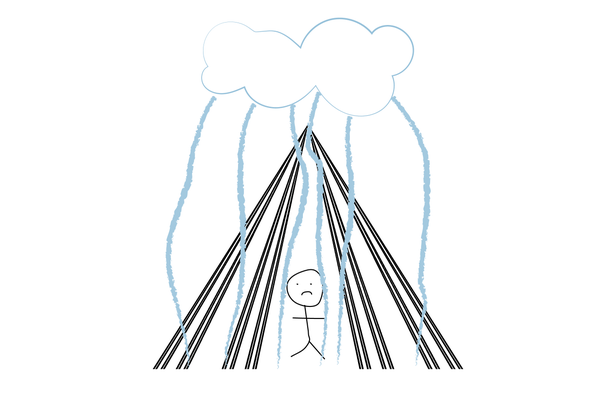
To construct Cantor’s leaky tent, sometimes called the Knaster-Kuratowski fan, you start by putting the Cantor set on the x-axis in the Euclidean plane and adding the point p=(1/2,1/2). Then you connect every point in the Cantor set to the point p with a straight line. At this point, we have a nice watertight tent.
Now we poke holes in it. There are two types of points in the Cantor set. Some, like 0, 1/3, and 1, are endpoints of removed intervals, and some, like 1/4, aren’t. We’ll consider these two types of points separately. For every point x in the Cantor set, we have a line connecting x to p. We’re going to modify those lines.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
If x is an endpoint, we’ll only think about points in the line that have rational y coordinates. If x is not an endpoint, we’ll only think about points in the line that have irrational y coordinates. Now each line from the Cantor set to p has been completely shattered.
Surprisingly enough, we aren’t left with just a mess of scattered points on the plane. Cantor’s leaky tent is connected.
It’s surprisingly hard to define what it means for a space to be connected, but I attempted to when I wrote about the topologist’s sine curve. Basically, a space is connected if we can’t find two sets A and B so that part of the space is in A and part of the space is in B and the spaces A and B don’t overlap. (We also need the technical condition that A and B are open, meaning around every point in each set, there is a blob that is also in the set. If you want more details, check out last month’s post.)
We poked a lot of holes in Cantor’s leaky tent, so it sure seems like it should be disconnected. If you’re trying to walk from a point in the Cantor set to the point p, you can’t do it. But somehow, that’s not quite enough.
The full proof that Cantor’s leaky tent is connected is pretty intense, so I’ll leave a link here for intrepid readers. The way I thought about it was to start by trying to put the tent into two separate open sets, A and B. The point p is special, so I tackled it first. It has to be in either set A or set B, so it might as well be A. Then because A and B are both open, there has to be a blob around p that is entirely within A. That blob has to contain points from all the lines, so there must be some transition points somewhere on some of those lines so some of the lines can have points in B. Pinning down exactly how that goes wrong is where the fun (and challenge) lies.
Cantor’s leaky tent is connected but just barely. The top point p is like a string hanging off a sweater: if you tug on it, the whole thing unravels. When we remove p, not only does the set become disconnected, it becomes as disconnected as possible. You can separate it into two disjoint pieces fairly easily: one of the pieces contains points with x coordinates less than 1/2 and one with x coordinates greater than 1/2. But it’s even messier: no two points in the new set, sometimes called Cantor’s teepee, are in the same connected piece. For any two points, you can always find a way to separate Cantor’s teepee into subsets that keep the points apart. Cantor’s Leaky Tent has just one piece, but Cantor’s teepee has infinitely many, and they’re just single points. If you’re interested in the lingo, p is called a dispersion point, and sets that don't have any connected pieces bigger than a point are called totally disconnected.
If Cantor’s leaky tent isn’t enough for you, there’s a related space called Cantor’s leakier tent that reverses the rational and irrational heights. Even though the sets of rational and irrational numbers are both infinite, there are more irrational numbers, so the sets aren't exactly interchangeable. In Cantor’s leaky tent, the countably many endpoints of the Cantor set are paired with the countably many rational points on the line to p. The leakier tent pairs the the countably many endpoints with the uncountably many irrational points and the uncountably many non-endpoints with the countably many rational points. This mismatch is enough to change some properties in a big way, and it demonstrates the importance of rigorous proofs to mathematicians. The argument I outlined above would seem to apply to either set, but while the leaky tent is connected, the leakier tent is not. For more on Cantor’s leakier tent, check out this Math Stackexchange thread.
Read about more of my favorite spaces: The Cantor Set Fat Cantor Sets The Topologist’s Sine Curve The Infinite Earring The Line with Two Origins The House with Two Rooms The Fano Plane The Torus The Three-Torus The Möbius Strip The Long Line Space-Filling Curves