This article was published in Scientific American’s former blog network and reflects the views of the author, not necessarily those of Scientific American
Ah, the Mandelbrot set. This famous fractal is a badge of honor for mathematicians. I have a poster of it hanging in my office, and you can buy t-shirts or jewelry depicting it at large math conferences and geek-oriented stores. What is there to say about the Mandelbrot set?
While the Mandelbrot set is instantly recognizable to a lot of math-interested people, I think many of them are hard-pressed to describe what it actually is. I certainly was a few years ago before I learned more about it for my post about fractal kitties. Many people know it’s self-similar and may even have watched videos zooming in on the beautifully intricate patterns there. But what is it?
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
As Holly Krieger explains in the above video, one way of defining the Mandelbrot set is by looking at how complex functions behave under repeated iteration. (One of my college math professors didn’t like it when we used the words “repeat” and “iterate” together because it was repetitive. I embrace that extra layer of meaning.) Here, “complex functions” are not necessarily terribly complicated; they’re functions that are defined on the complex numbers, numbers that have the form a+bi where a and b are garden-variety real numbers and i is √-1, often slandered as “imaginary.”
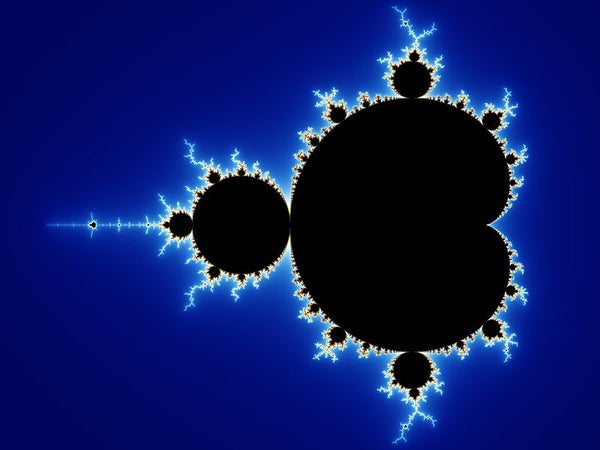
The functions in question have the form f(z)=z2+c, where c is a complex number. For each c, we seed the function by plugging in 0 as the initial value for z. Then we take whatever we get out and plug it back into the function. As we iterate the function, one of two things can happen: either the iterates get larger and larger in general, moving further and further away from 0, or they stay close, possibly bouncing around the area wildly but never getting very far from 0.
The values of c for which these iterates stay close to 0, colored black in the picture at the top of this post, make up the Mandelbrot set. The other points are colored based on how quickly their iterates run away to infinity.
Aside from its inherent beauty, the Mandelbrot set has a few mathematically fascinating properties. Its complicated structure demonstrates what mathematicians mean by chaos: points very close to each other can have dramatically different behavior, and around the edges of the set, it’s basically impossible to predict whether a point is going to go one way or another. It also has surprising and interesting connections to other fractals called Julia sets. You can learn more about them in this Plus Magazine article or this Numberphile video.
Mathematicians are still trying to solve some of the mysteries of the Mandelbrot set. In the early 1980s, Adrien Douady and John Hubbard proved that it was connected; in other words, that there aren’t tiny gaps separating any bulbs of the set from any other ones. Mathematicians still don’t know whether the Mandelbrot set is locally connected. The exact definition of this property is a little technical, but the idea is that if you zoom in on any point in the set, does it eventually look like a nice connected little blob? (The topologist’s sine curve is another space that is connected but not locally connected, though I didn’t mention that property when I wrote about it a couple years ago.) Mathematicians know that the Mandelbrot set is locally connected at a lot of its points, but it’s not known yet whether the entire thing is.
As far as I know, the Mandelbrot set itself doesn’t really have any practical applications, though fractals in general definitely do, but it bears a remarkable resemblance to a horseshoe crab, and that majestic creature has magical baby blue blood with lifesaving medical applications.

Thank you, my prehistoric friend. Credit: Tony Alter Flickr (CC BY 2.0)
Read about more of my favorite spaces: The Cantor Set Fat Cantor Sets The Topologist’s Sine Curve Cantor's Leaky Tent The Infinite Earring The Line with Two Origins The House with Two Rooms The Fano Plane The Torus The Three-Torus The Möbius Strip The Long Line Space-Filling Curves The Wallis Sieve Two Tori Glued along a Slit The Empty Set The Menger Sponge The Connected Sum of Four Hopf Links Borromean Rings The Sierpinski Triangle Lexicographic Ordering on the Unit Square The SNCF Metric