This article was published in Scientific American’s former blog network and reflects the views of the author, not necessarily those of Scientific American

A wearable, knitted (5,3) torus knot. Image: sarah-marie belcastro.
Step aside, infinity scarves, you aren't infinite at all. The (5,3) torus knot cowl is where it's at.
For me, one of the highlights of January's Joint Mathematics Meetings was the mathematical fiber arts session. You can view a slide show I put together from the session here. During the session, co-organizer sarah-marie belcastro gave a talk about her beautiful knitted torus knots and links. I included a picture of them in the slide show but wanted to share a few more details about them here on the blog.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
In mathematics, a knot is basically what it is in the real world. You take a length of string, tie it up somehow, and attach the free ends together so it doesn't have a starting or stopping point. (I wrote about knots in a blog post last year, in case you'd like to explore them more.) A link is any number of knots put together. They might just be stuck next to each other, or they might be entwined in some interesting way.
A link with three components. This configuration is called Borromean rings, and it is interesting because no two circles in the picture are linked with each other, but when all three are put together, you can't pull them apart. Image: Public domain, via Wikimedia Commons.
Torus knots and links, the subject of belcastro's talk, can be made by wrapping a string around a torus, or a donut. You can think of a torus as the shape you get when you sweep a circle around another circle as in the diagram below.
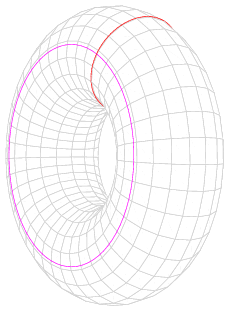
A torus is what you get when you drag the red circle along the pink circle. Image: Fropuff, via Wikimedia Commons.
We can think of the red circle and the pink circle, the circles that wrap through the hole or around the hole, respectively, as the two main "directions" on the torus, analogous to latitude and longitude on a sphere.
A torus knot or link is defined by how many times it wraps around the two main directions. A (p,q) torus link* wraps p times through the center (along the red circle) and q times around the hole (along the pink circle). If we use a negative number instead a positive one for p or q, the description is the same, but the knot or link spirals around the torus in a different direction. For more illustrations of torus knots, check out the Knot Atlas.
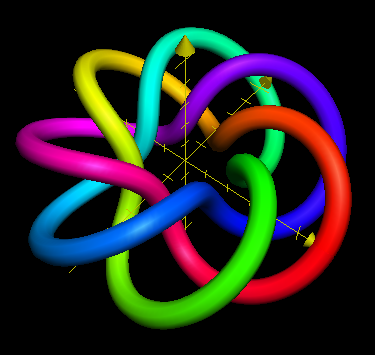
A (-7,3) torus knot rendered in 3D. It wraps 3 times around the hole of the torus and 7 times through the hole but twists around in a different direction from the (7,3) torus knot. Image: Michiel Sikma, via Wikimedia Commons.
Any two integers p and q can define a torus link. If the numbers don't share a common factor, the link only has one piece, so we call it a knot. If they do share a common factor, the link has more than one piece, like this (4,2) torus link. (The number of pieces is the same as the largest common factor that p and q share.)
-Torus_Link.png?w=500)
A (4,2) torus link has two components. Image: Public domain, via Wikimedia Commons.
Belcastro is both a mathematician and a knitter, and she has become adept at combining the two subjects. She uses mathematics to understand and guide her knitting and knitting to visualize complicated mathematical objects. A few years ago, belcastro started knitting tori using contrasting yarn to illustrate torus knots on them.

A (5,3) torus knot in light green on a background torus of dark green. Image: sarah-marie belcastro.
As she continued to use knitting to explore mathematics, she thought about getting rid of the torus and just knitting the knots by themselves. Of course, one way to do it would be to knit a skinny rectangle, tie it in a knot, and sew the ends together. But that would just be too easy! Instead, belcastro wondered if she could knit so that the knot would be intrinsically created by the knitting instead of being added after the fact. One day, she tied her circular knitting needles (these are basically two points with a long flexible wire connecting them) in a knot and ended up with a knitted trefoil knot.

A trefoil knot. Image: Public domain, via Wikimedia Commons.
The trefoil is the simplest knot other than a circle, and it is also a (3,2) torus knot. After successfully knitting the trefoil, belcastro wondered which other torus knots and links she could create and eventually discovered a recursive algorithm for wrapping the needles around correctly to knit some torus knots. (Specifically, her procedure works for (nk+1, k) torus knots and ((n+2)k-1,k) torus knots, for any integers n and k.)

A knitted (15,5) torus link. Image: sarah-marie belcastro.
To create torus links with multiple pieces, belcastro knits one knot in the link first using her algorithm and then uses it as the base for the next component of the link, which she also creates using her wrapping algorithm. She keeps doing this until she has as many pieces as she needs. Because she wraps the needles around the previous components to knit each subsequent knot, the finished product has all the knotty-ness and linky-ness built in; there's no need to join up the ends after the fact.

Knitted torus links. From left to right, they are (4,2), (6,4), and (8,6) torus links. Each link has two components. Image: sarah-marie belcastro.
Belcastro says that at the end of knitting a torus link, the project looks like a total mess. But she has learned to have faith that when she takes it off the needles and carefully flattens it out, she will end up with a beautiful, correctly executed torus link. She usually makes knots for their mathematical properties alone, but recently she discovered that with some small tweaks, her (5,3) torus knot would make a beautiful cowl. Wearable math for the win!
*Torus knots can be labeled with either direction first. Wikipedia adopts the convention that "around the hole" comes first, but belcastro adopts the convention that "through the hole" comes first. The original version of this post used both conventions at different points. I have updated the post to be consistent, using the convention belcastro uses. If you end up surfing over to the Wikipedia page, keep in mind that its torus knots are labeled using the opposite convention.