Sometimes it seems like in the world of interesting mathematical examples, all roads lead to the Cantor set. Or maybe it’s more like all roads start from the Cantor set. My first “favorite space” post here is on the Cantor set, and I wrote it because I wanted to write about a function that is based on the Cantor set, and I felt like I was trying to cram way too much into one article. Our most recent My Favorite Theorem episode features the Cantor set and a related space, Cantor dust, in an important way. It feels like everywhere I turn, I run into the Cantor set.

Seven steps in the middle-thirds construction of the Cantor set. The first line is a solid interval. In the next line, the middle third has been removed, and in the next line, the middle thirds of the remaining intervals, and so on. Credit: 127rect Wikimedia

I feel a little weird saying the Cantor set, though, because for the space I’m writing about today, it makes more sense to call it a Cantor set. The standard middle-thirds construction, which you can read about here, is just one way to think about a space with three specific mathematical properties. Any space with these properties can be called a Cantor set, and in some sense, there's nothing special about the middle-thirds construction. It's just a convenient way to arrive at a space with a few special properties.

Here are the rules.

  • First, the space must be totally disconnected, which means that no two points are in the same “chunk” of the space. A space like the circle would be connected because all the points are in the same chunk. A space that looks like two interlinked circles would be disconnected but have two connected parts of one circle each. For the middle-thirds Cantor set, though, any two points have a certain finite distance from each other, and the construction guarantees that there are no segments of that length that are left in the set.
  • Second, the space must be compact, which for now means it’s entirely contained within a certain finite radius, and contains all points in the set that approach some limit, appropriately called limit points. The classic example is that the interval (0,1), all the points greater than 0 and less than 1, is not compact because you can imagine a sequence of points approaching the limits of 0 and 1, but those points aren't contained in the set. On the other hand, the interval [0,1], which contains the endpoints 0 and 1, is compact.
  • The third condition is sort of the opposite of the second one. In a compact space, every limit point must be part of the space. Here, every point in the space must be a limit point. Another way to think about it is that there are no points that are far away from all other points in the set. Every point has nearby neighbors. This property is called being a perfect set.

All totally disconnected, compact, perfect sets are Cantor sets, whether they come from a middle-thirds construction or not, and all Cantor sets are homeomorphic to one another, which means there’s a way to continuously mush one of the spaces into the other space and back again.

I write this rather long prelude to any real description of Antoine’s necklace because it’s a Cantor set that is not immediately recognizable as equivalent to the middle-thirds construction, and I don’t want to get hung up on trying to unearth the middle-thirds construction here. Antoine's necklace is a Cantor set—in fact, maybe I should even say Antoine's necklaces are Cantor sets because there are an infinite number of necklaces we can make, all of which are similar in some ways and distinct in some ways. This construction was first described by French mathematician Louis Antoine in a 1921 paper, but I consulted a College Mathematics Journal article by Beverly Brechner and John Mayer for this post.

We can build Antoine's necklace in stages. We start with a solid torus, a donut shape, sitting in three-dimensional space. This is level 0 of Antoine’s necklace.

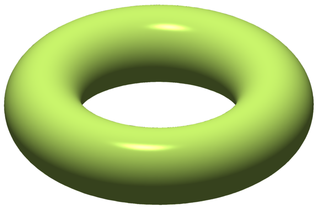
A solid torus, or donut, shape. Credit: Oleg Alexandrov Wikimedia

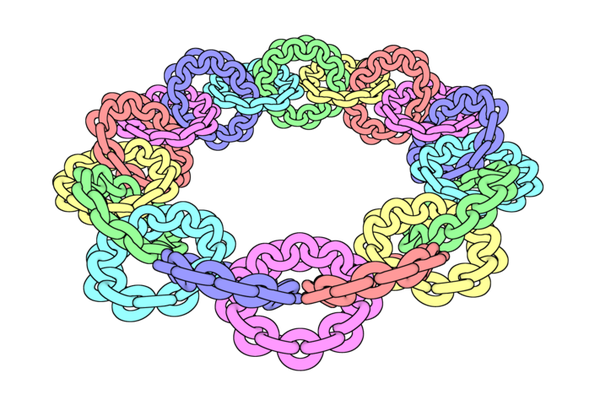
Now we replace the torus with a chain of smaller tori sitting entirely within the first torus to get level 1 of the construction. How many smaller tori? However many you want! Blacklemon67, the generous soul who uploaded a picture of Antoine's necklace to Wikimedia Commons, chose 18, so let’s go with that.

A multicolored chain made of 18 links.
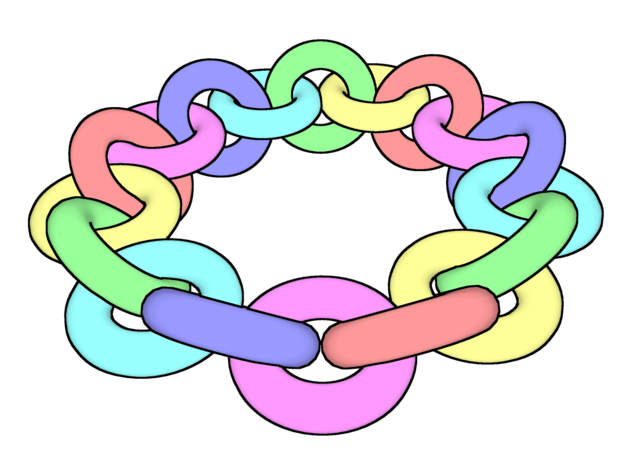
Iteration 1 of the construction of Antoine's necklace. It looks like, well, a necklace, specifically a necklace chain. Credit: Blacklemon67 Wikimedia (CC BY-SA 3.0)

And now we replace each torus in the chain with another chain of smaller tori, in this case 18 again.

A chain of 18 links in which each link is made of 18 smaller links
Credit: Blacklemon67 Wikimedia (CC BY-SA 3.0)

You might be sensing a pattern. At each stage, we replace every torus with a chain of smaller tori, and we do that forever.

To verify that Antoine’s necklace is a Cantor set, we have to check that it satisfies the three conditions above. I wouldn't want to spoil your fun by delving into the details here, but you can check the Brechner and Mayer article for more information.

Antoine’s Necklace illustrates a subtle fact about the ways in which spaces can be equivalent in math. Homeomorphism is one version of equivalence. All Cantor sets are homeomorphic, which means there’s a way to map the points of one of our Antoine’s necklaces to the points of the Cantor set in a continuous way so that the backwards version, or inverse, of the function, from the Cantor set to Antoine’s necklace, is also continuous.

But there’s another, stronger definition of equivalence we could ask for. We could request this continuous function from one mathematical object to another with a continuous inverse also be a homeomorphism of the ambient space around the two objects, which basically mean it doesn't just have to be nice to the mathematical object but to the entire universe the object lives in. This is called ambient homeomorphism. In the case of the Cantor set and Antoine’s necklace, we can’t do that. And it’s not just because we usually think of the Cantor set as living in two dimensions and Antoine’s necklace as living in three. We can also stick the middle-thirds Cantor set in three dimensions and still run into a problem. In fact, if we had picked numbers other than 18 at each level of the construction, the Antoine’s necklaces we obtained would not be equivalent in this stronger sense either. Every collection of choices we can make about the number of tori to include at a particular step gives us a new necklace. We can see that these spaces don’t have this stronger ambient homeomorphism by looking at what happens around them.

One important way to study a space’s properties is by looking at how loops in the space behave. You can imagine drawing a loop outside of the middle-thirds Cantor set (sitting in three-dimensional space) and convince yourself you can free the loop from the space by sliding it around. The Cantor set won’t be able to obstruct the loop. But it’s harder to imagine that with Antoine’s necklace. Now just because it’s harder to imagine doesn’t mean we can't do it, but in this case, it's true. Start with a loop in the space around Antoine’s necklace that goes through the center of the solid torus in the first step, as if you were stringing the necklace itself onto another necklace. We can never completely disentangle the loop from Antoine's necklace. As Brechner and Mayer put it, it’s like the necklace is made of tiny beads that aren't being held together by any kind of string but nonetheless can't fall apart.

I really like Antoine's necklace because in some ways it’s child’s play. I remember how much I liked making loops out of loops that were made out of loops when I was a kid. But you can let the child's play lead you into discovering some subtle, deep mathematics.

Thanks to UNC Asheville math professor Mark McClure for telling me about Antoine’s necklace and pointing to the Brechner and Mayer article I found so helpful. His website has a model of Antoine's necklace you can play with and drag around and a lot of other fun math toys, including this Julia set explorer I mentioned in my post on the Douady rabbit.

Read about more of my favorite spaces:
The Cantor Set
Fat Cantor Sets
The Topologist’s Sine Curve
Cantor's Leaky Tent
The Infinite Earring
The Line with Two Origins
The House with Two Rooms
The Fano Plane
The Torus
The Three-Torus
The Möbius Strip
The Long Line
Space-Filling Curves
The Wallis Sieve
Two Tori Glued along a Slit
The Empty Set
The Menger Sponge
The Connected Sum of Four Hopf Links
Borromean Rings
The Sierpinski Triangle
Lexicographic Ordering on the Unit Square
The SNCF Metric
The Mandelbrot Set
Fatou's Pancake
The Pseudosphere
The Douady Rabbit
The Poincaré Homology Sphere
The Kovalevskaya Top
A 6-Holed Torus
The Real Projective Plane
The 1-Dimensional Sphere
The Loch Ness Monster
The Koch Snowflake
The Bicylinder
The Catenoid
SO(3)
The pseudo-rhombicuboctahedron 
The Moser spindle
The Witch of Agnesi