This article was published in Scientific American’s former blog network and reflects the views of the author, not necessarily those of Scientific American
I don't often write about my own research on these pages, but recently I've been looking at ways to bring together what we are learning about exoplanets with our ongoing guesswork about the origins of life.
In astrobiology and exoplanetary science the property of 'habitability' is a key area of study. Put simply, the question of habitability is whether or not an environment (such as that on the surface of a planet) is capable of sustaining life. In other words habitability does not necessarily mean inhabited - but it's a good way to try to find places in the universe that could be. So astronomers and planetary scientists are trying to evaluate whether physical conditions on different worlds fall into a range where liquid water exists, and whether or not biologically useful chemical energy and chemical components may be present.
Of course there are all kinds of caveats to habitability. The modern Earth is only habitable to organisms like humans because of the prior existence of other organisms that have oxygenated the atmosphere, and otherwise sculpted the planet to its present state. And the conditions of habitability today are not necessarily compatible with the conditions that gave rise to life - in other words, the origins of life may require something altogether different than 'standard' habitability.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
But the study of life's origins is a hugely challenging and fraught topic (something I've written about before). Quite often research on origins has been undertaken by isolated scientists, and quite often the approaches have involved the development of complex 'stories' about the steps necessary to go from an abiotic world to a living world.
It may well be that this is how we'll end up reconstructing the origins of life on Earth. But a 'story' for Earth may not help us very much when it comes to estimating the probability of life arising elsewhere in the universe.
There are a couple of ways we can try to answer that puzzle. The first approach is what astrobiology is aiming for: to find as many instances of living systems in the cosmos as possible and use those to constrain the rate at which life emerges, or the probability of life happening in a statistical sense. The second approach is to figure out whether life is actually a genuinely universal phenomenon - in the sense that the thing we call life might use a variety of chemical and physical toolkits (or even virtual toolkits within machines), and follow a variety of pathways to come into existence.
Success with either approach would be a big deal and would help us estimate the rate at which life pops up across the cosmos. Right now though we don't have very much information to work with.
Back in 1961 Frank Drake introduced his now famous Drake Equation in an effort to bring focus onto the various factors that must be determined in order to compute how many communicative civilizations might exist in our galaxy. It's been a very useful tool for summarizing the extent of our ignorance to date.
But can we write a similar kind of equation to bring focus onto the factors that go into computing just how often life might arise in the cosmos?
I think we can, and that's what myself and Lee Cronin have done in a new paper just published in the Proceedings of the National Academy of Sciences. In its simplest form the equation looks like this:
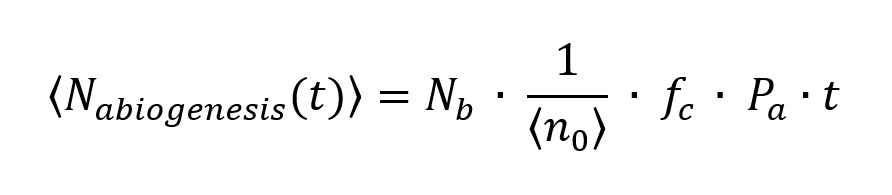
On the left is an expression for the average number of origin of life 'events' that would occur on a planet during a certain length of time (t) - if you could average over a whole bunch of similar planets. An origin of life event is something that the equation doesn't need to define very exactly. The event could literally be the moment that a living entity (like a cellular lifeform) switches on, or it could be a whole sequence of phenomena separated by space and time which nonetheless result in the initiation of a living system (which need not be neatly localized either).
The terms on the right hand side are (in order, left to right): the number of potential building blocks (like atoms or molecules) for living systems on a planet, the reciprocal of the average number of building blocks that can make a living system (e.g. a bacterium), the fractional availability of those blocks (i.e. those not locked up in inaccessible states or conditions), the probability (Pa) of an abiogenesis (origin) event per set of necessary building blocks per unit time, and finally the length of time in question. Our paper (freely available here) fills in the details surrounding these factors - including ways in which the fractional availability of building blocks (fc) can be expanded out into all kinds of new (measurable) factors.
The bottom line is that we're proposing that you can sneakily hide all the unknown complexity of abiogenesis (an origins of life event) inside these simple terms - especially the probability of assembly Pa. But at the same time, the neat thing about the equation is that it explicitly links the microscopic phenomena involved with life (the building blocks that could be atoms or molecules) to the macroscopic properties of a planet (or any environment for that matter), through the number of building blocks and their availability (how big a planet is, what it's made of, and what thermal state it's in). In other words, the equation provides motivation for making specific measurements of planetary properties and histories (as well as running specific lab experiments).
The left hand term, Nabiogenesis can also be constrained by astrobiologists who find life elsewhere, and can even now be given some range of values by careful Bayesian analyses that take our ignorance into account.
All of which means that even today we can make some very crude estimates of what that root (microscopic) probability of assembly is, Pa. In our paper we propose that it might be as small as about 10-33 for the Earth. But the sheer size of the planet, combined with millions of years of chemical 'searching' some 4 billion years ago, can overcome that low likelihood to result in at least one successful abiogenesis event.
We also realized that by conceptualizing the origins of life this way we may need to take into account phenomena that can stir the chemical pot. For example, Earth and Mars seem to have exchanged significant amounts of material over their history, and especially in the early solar system when each world was being bombarded by asteroids and spraying out surface rocks into space.
It might be difficult for viable organisms to be transferred between planets (types of panspermia), but probably a lot easier for different chemical toolkits to be exchanged. In other words, instead of a single planetary chemical incubator you could have had two - each running a different set of experiments and sharing the results.
Our equation lets us easily add that factor, and in principle it could result in an exponential boost in the chemical 'search space', and could represent the difference between life occurring on a world like the Earth or not. It could therefore also mean that the underlying, non-amplified value of Pa is almost indistinguishable from zero!
This idea suggests that the final odds of 'Earth-analog' worlds harboring life might vary wildly from system to system, depending on whether a similar exchange of material amplified the chemical complexity or not.
Of course all of this is still hugely speculative. But I think with this Origins version of a Drake equation (and gosh, the Scharf-Cronin equation has a nice ring to it!) we might have a new entry point to the problem, a slightly different way to approaching the questions surrounding origins and, critically a way to begin quantifying and testing those questions.
