This article was published in Scientific American’s former blog network and reflects the views of the author, not necessarily those of Scientific American
When you look through a kaleidoscope, the scene in front of you becomes a mosaic of tiles. My mathematical art is similar, but with a twist: it turns the textures and colors of the world into a pattern, but without the rigidity of mirrors. This work—detailed in my new book, Creating Symmetry: The Artful Mathematics of Wallpaper Patterns—is recognizably mathematical, but with a wild variety of rhythm, and this makes it symmetric, yet organic.
For instance, consider my “Scientific American Sunset,” below, made from a photograph of a surfer with the Scientific American logo set over the sun. On one hand, we can see the surfer and the sunset, and we can read the logo; on the other, the repeating rhythm signals that this is unmistakably a mathematical pattern.
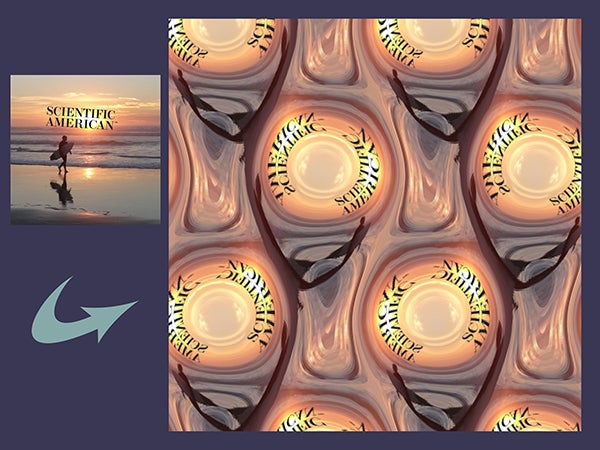
Credit: Frank A. Farris
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
When we see the repeats in two different directions, our minds naturally extend the pattern past the edges of the image. We accept that what we see is a small swatch of a pattern that continues indefinitely on all sides. In this way it is like wallpaper: a pattern that repeats forever along two different axes.
Wallpaper patterns like these are called “pg” by the International Union of Crystallographers. This name draws our attention to the additional symmetries of the image, beyond the simple repetitions. The g indicates the pattern’s glide symmetry: if you flip across certain vertical axes and then slide the image up or down by half the repeat length, the pattern is unchanged. Look for the two visually different glide axes in this image: one along the dark band of ascending curvy surfers, the other touching the surfboard tips. See the “flip and slides”?
The remarkable fact about wallpaper is that there are exactly 17 types. No matter how creative you may be as you introduce symmetries into a pattern that repeats indefinitely along two different axes, you will inevitably create one of these types. Since these patterns underlie the structure of crystalline matter, this says something about the nature of reality, not just the nature of mathematics.
Knowing the theorem about the 17 wallpaper types is just the sort of thing that makes many of us feel that mathematics is discovered, not invented.
How was this image made? I adapted methods from mathematical tools called Fourier series and complex analysis to create complex-valued wave functions that have a given, selected symmetry. For the image above, I used wave functions locked together in just the right way to create the glide symmetry. The complex value of the wave function at any given point is enough to locate a pixel in the photograph, whose color values are used to color the point.
The rest of the process is experimental. Changing the amplitudes and complex phase of the individual waves alters the image dramatically. This one was a particular challenge, because I wanted the Scientific American logo to be legible. I have created thousands of these images before, but have only been truly pleased with a few dozen of them, among which I’m happy to count this one.
The same techniques can be used to create symmetry in many other categories. If you want repetition along only one axis, I can show you “frieze functions” that would create art for a decorative pattern for a band at the top of a wall. For patterns without any translational symmetry at all, I have “rosette functions,” like this one:

Credit: Frank A. Farris
Here we see the Scientific American logo, a Paleolithic stone monument, and Sierra wildflowers propagated through a rotor shape that appears the same upside down as right-side up: two-fold rotational symmetry. The juxtaposition of a nature photo with a creation of human industry seemed appropriate for Scientific American.
A tidbit for mathematicians: The function depicted here is a short Laurent series for a meremorphic function where the highest power is four and the lowest power is negative six. By the argument principle, you should be able to see 10 zeroes of this function, which occur when the function selects a pixel that lies between the NT and RI of Scientific American in the center of the collage.
For those who tire of Euclidean plane symmetries, my book explains how to create images with polyhedral symmetry, as well as certain non-Euclidean symmetries in the Poincaré Upper Halfplane. I also explain the various kinds of color symmetry, which also places mathematical limitations on the number of pattern types it is possible to create.
There is no reason not to apply these ideas to 3D printing, but I have so far not done so. Perhaps I’m holding out for the incentive of full-color printing. Similarly, I have produced only very primitive animated images—vibrating wallpaper!
My techniques for making mathematical art from photographs offer limitless variety. Surely I should not be the only person using them. I have projects underway to improve my own tools, but there is room for broader participation. Perhaps someone reading this blog will become involved in the next stage of putting these tools into the hands of artists. All the details you need are in the book!